1234
of 4

Solving Exponential Equations

So far, we've been solving equations like
 

2x - 5 = 7andx^( 2 ) - 3x - 10 = 0

(a linear equation)

 

(a quadratic equation)

But, NOW the unknown is going to be in the exponent!

Check it out:

Solve  5 = e^( 7t )

We need a way to get to the t...  We HAVE to solve for Mr. t.  I pity the fool who can't solve Mr. t !  Pity the fool!  (Sorry for the lame A-Team joke.)

5 = e^( 7t )

Who is in the way?

e!

How can we ditch that e?  Can you think of anything that will undo it?

The natural log!  He's an e killer!

Just remember... 
Anything we do to one side of an equation,
we have to do to the other!

 

So...  take the natural log of each side:

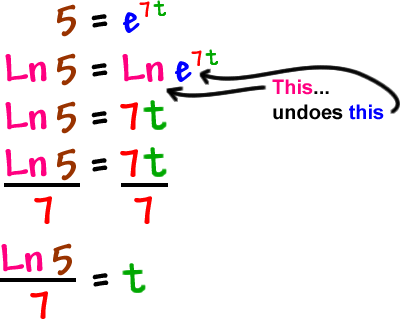
5 = e^( 7t ) ... Ln( 5 ) = Ln( e^( 7t ) ) ... Ln( 5 ) = 7t ... Ln( 5 ) / 7 = 7t / 7 ... Ln( 5 ) / 7 = t ... the Ln undoes the e

So,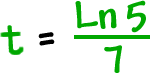t = Ln( 5 ) / 7

You can pop this into your calculator...

t = approximately .2299

(Four decimal spots is OK.)